C++ Program For Bisection Method

The bisection method is a root finding numerical method. Given a function the bisection method finds the real roots of the function. In this article you will learn to write a program for bisection method.

Problem Definition

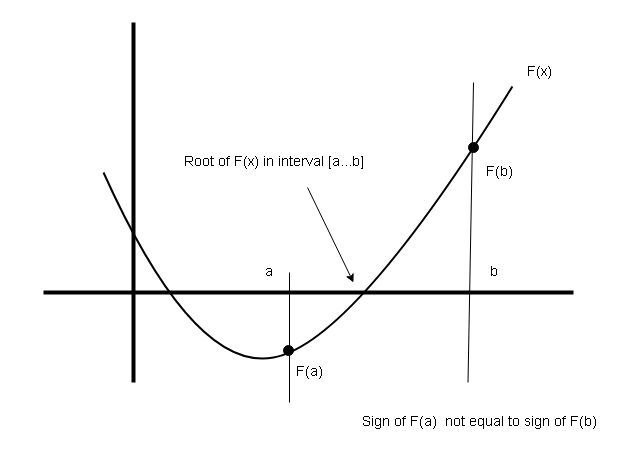
The bisection method find the real roots of a function. Suppose you are given a function and interval [a…b] the bisection method will find a value such that .

Read More: Bisection Method

The value lies between the interval [a…b]. There few rules to find roots using bisection method.

• The sign of sign of .
• The function must be continuous.
• Cuts the interval into 2 halves and continue searching smaller half for roots.
• Keep cutting the interval into smaller and smaller halves until interval is too small.
• The final value at the smallest interval is the root.

Program Code – Bisection Method

/* C++ Program to evaluate a function using Bisection Method

Function: F(x) = x^3-4x-9

File Name: BisectionMethod.CPP

Author: NotesforMSc
*/

#include "iostream.h"
#include "stdio.h"
#include "conio.h"
#include "math.h"
#define MAX 20


void main()
{
   int i;
   double a, b, m,fa,fm;

   //Initialize variables

   fa = fm = 0.0;
   m = 0.0;
   clrscr();

  cout << "\n\t Enter initial value:";
   cin >> a;
   cout << a << endl;
   cin >> b;
   cout << b << endl;
   cout << "\n\n\t\t SOLUTION BY BISECTION METHOD"<< endl;

   // Bisection Method



  i = 1;

   while(i < MAX)
   {
      m = (a + b)/2.0;
      fa = (a * a * a) - 4 * a - 9;
      fm = (m * m * m) - 4 * m - 9;

      //Check the smaller intercal

      if(fa < 0 && fm > 0) {
	 b = m;
	 }
      else {
       a = m;
       }
      i++;

      }

      //Print the Results

      cout << "\n\t Result";
      cout << "\n\t Root is" << " " << m << endl;

      getch();

   }

Output – Bisection Method

            Enter Initial Value: 2
2
3
3
                       SOLUTION BY BISECTION METHOD
                 
              Result
              Root is 2.70653

References:-

Shun Yan Cheung () The Bisection Method, Available at: http://www.mathcs.emory.edu/~cheung/Courses/170/Syllabus/07/bisection.html (Accessed: 6/19/2019).

University of Waterloo, Department of Electrical and Computer Engineering () Topic 10.1: Bisection Method (Examples), Available at: https://ece.uwaterloo.ca/~dwharder/NumericalAnalysis/10RootFinding/bisection/examples.html (Accessed: 6.119/2019).