The graph of equation has symmetry along the x-axis or y-axis. In this article, the symmetry of a graph of function is defined in terms of even and odd function.
Prerequisite for this articles is listed below.
Even Function
Let’s understand the even functions first. The function 
is even function, if for every x in domain of 
.
f(-x) = f(x)
For example,
Let be a function where
f(x) = x^2
The function will square any input value for 
and output 
. Let us put some values for , see the following table of values.
| x | y = f(x) | point |
| -2 | 4 | (-2, 4) |
| -1 | 1 | (-1, 1) |
| 0 | 0 | (0, 0) |
| 1 | 1 | (1, 1) |
| 2 | 4 | (2, 4) |
The table shows that any 
values is equal to .
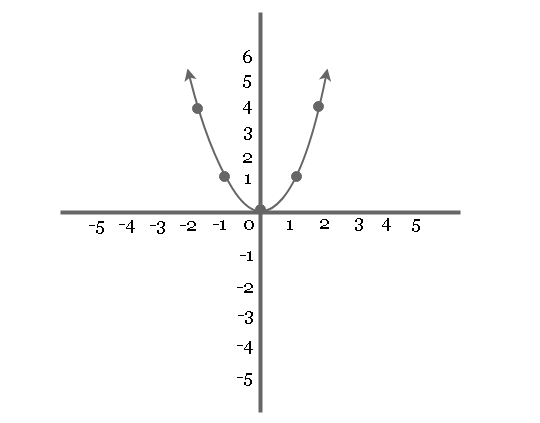
The graph of even function is symmetric along the y-axis.
Odd Functions
The odd function is different from even function in terms of symmetry of graph of the function. The function is odd function if for every in the domain of .
f(-x) = -f(x)
Consider the following table of points for the function 
.
| x | y = f(x) = x + 1 | point |
| -3 | -2 | (-3, -2) |
| -2 | -1 | (-2, -1) |
| -1 | 0 | (-1, 0) |
| 0 | 1 | (0, 1) |
| 1 | 2 | (1, 2) |
| 2 | 3 | (2, 3) |
The graph of odd function shows that 
is 
.
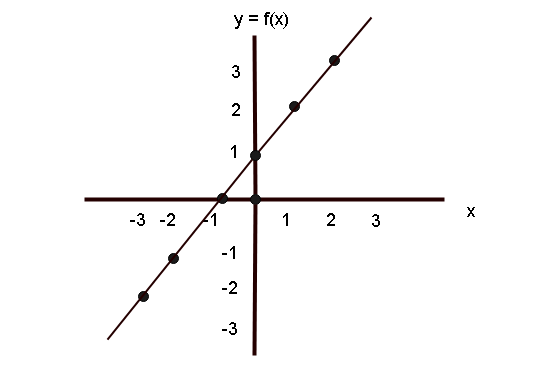
The graph of odd function is symmetric along the y-axis.
